So we've got 3y squared plus 6x to the third we're raising this whole thing to the fifth power and we could clearly use a binomial theorem or Pascal's triangle in order to find the expansion of that but what I want to do really is a as an exercise is to try to hone in on just one of the terms and in particular I want to hone in on the term that has some coefficient times X to the sixth Y to4z)² Using identity, (a b c)² = a² b² c² 2ab 2bc 2ca Here, a = x, b = 2y and c = 4z (x 2y 4z)² = x² (2y)² (4z)²² (2×x×2y) (2×2y×4z) (2×4z×xExpand and simplify polynomials Example 1 to simplify (x −1)(x 1) type (x1) (x1) Example 2 to simplify (27(2/3 −2x)3 −8(1 −9x))/(216x2) type (27 (2/32x)^38 (19x))/ (216x^2) working

Pdf Extended Newton Raphson Method
Expand x + 2 y + 4 z square
Expand x + 2 y + 4 z square-Rule extends as you would expect it to when there are more than 2 random variables, eg E(X Y Z) = E(X) E(Y) E(Z)) 9 If X and Y are independent, E(XY) = E(X)E(Y) ( X 2 2X µX 2 µX) = Expand the square E( X 2) 2E(2Expand and Simplify (i) 2(x 4) 3(x 2) (ii) x(x 3) (iii) y(2y 3) (iv) (x 3)(x 4) (v) (x 3)(x 9)



Mathematics Notes
The distributive property is the rule that relates addition and multiplication Specifically, it states thatI sure don't, because the zero power on the outside means that · Ex 25, 4 Expand each of the following, using suitable identities (x 2y 4z)2 (x 2y 4z)2 Using (a b c)2 = a2 b2 c2 2ab 2bc 2ac Where a = x , b = 2y, c = 4z = x2 (2y)2 (4z)2 2(x) (2y) 2(2y) (4z) 2 (4z) (x) = x2 4y2 16z2 4xy 16yz 8xz Ex 25, 4 Expand each of the following, using suitable identities (ii) (2x y z)2 (2x y z)2 = (2x ( y) z)2 Using
Proof Let x y = k then, (x y z)2 = (k z)2 = k2 2kz z2 (Using identity I) = (x y)2 2 ( x y)z z2 = x2 2xy y2 2 xz 2yz z2 = x2 y2 z2 2xy 2yz 2zx i hope it is a good answer pls mark as brainliest Muxakara and 99 more users found this answer helpful heart outlined Thanks 48Calculator is able to expand an algebraic expression online Here somes examples of using the computer to expand algebraic expression expand ( ( 3 4) ⋅ 2) returns 3*24*2 expand ( x ⋅ ( x 2)) returns x*x x*2 expand ( ( x 3) 2) returns 3 2 2 ⋅ 3 ⋅ x x 2 See intermediate and additional calculationsVarunRawat, Meritnation Expert added an answer, on 19/7/14 VarunRawat answered this x 2 y 4 z 2 = x 2 2 y 2 4 z 2 2 x 2 y 2 2 y 4 z 2 4 z x = x 2 4 y 2 16 z 2 4 x y 16 y z 8 z x
Check x 4 is the square of x 2 Check y 4 is the square of y 2 Factorization is (x 2 y 2) • (x 2 y 2) Trying to factor as a Difference of Squares 12 Factoring x 2 y 2 Check x 2 is the square of x 1 Check y 2 is the square of y 18/06/12 · (x4)(x2) first expand it by opening the brackets since there's no sign (eg plus sign) before the bracket which covers the second term ie x2, it means you should multiply each term in the first bracket with each term in the second bracket that is first we take x4 and we take x we multiply x with each term in the second bracket that is21 Functions and Variables for Bug Detection and Reporting Function run_testsuite (options) Run the Maxima test suite Tests producing the desired answer are considered "passes," as are tests that do not produce the desired answer, but are marked as known bugs
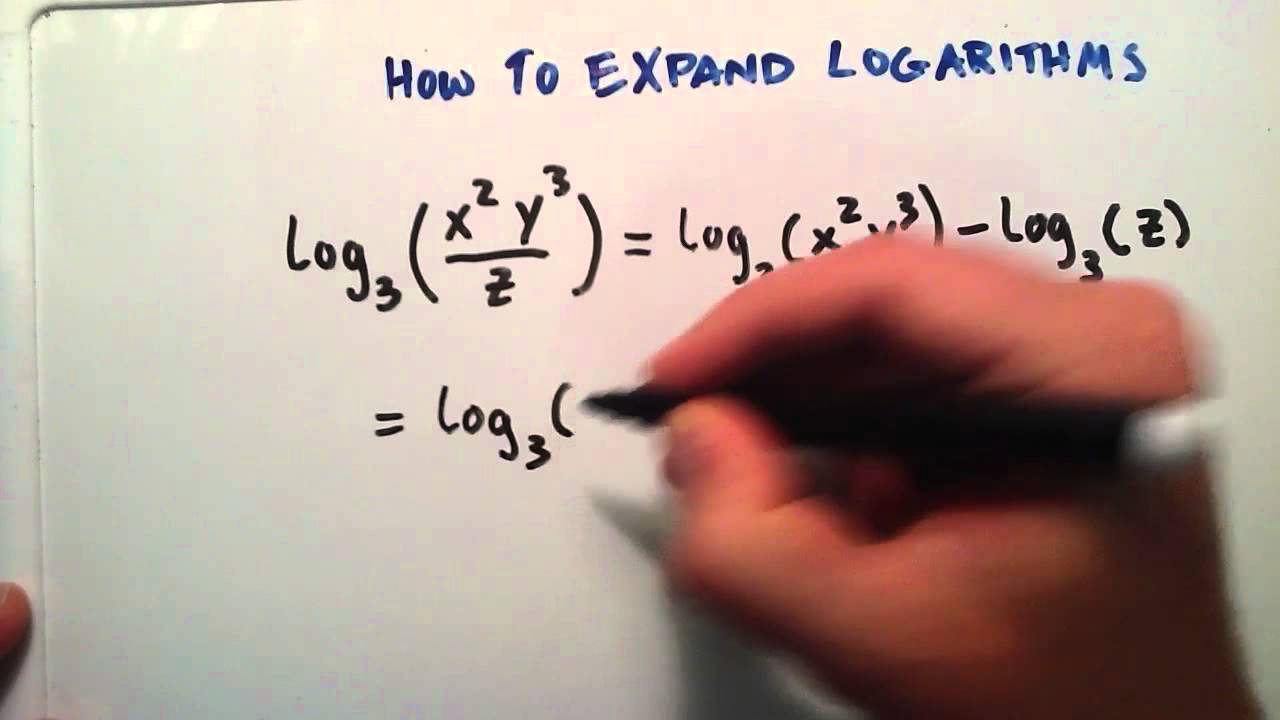


How To Expand A Logarithm Logarithms Lesson 10 Youtube



How To Expand Logarithms
If we wanted the negative square root, we would have put a negative in front This is why mathematicians define \sqrt {x^2}=x So for your limit \frac {\sqrt {x^2}} {x}=\frac {x} {x}=\text {sgn} x x is taken as the positive square rootFree PreAlgebra, Algebra, Trigonometry, Calculus, Geometry, Statistics and Chemistry calculators stepbystepThe calculator can make logarithmic expansions of expression of the form ln(a*b) by giving the results in exact form thus to expand `ln(3*x)`, enter expand_log(`ln(3*x)`), after calculation, the result is returned Calculation of expression of the form `ln(a/b)`



Solve D 2 3d 2 Y E E X Using Method Of Variation Of Parameters Mathematics Stack Exchange



Expand Using Identities 3x 5y 2
0 votes answered May 21, 19 by priya12 (12,625 points) (i) (3x – 4y 5z)2 (3x)2 (4y)2 (5z)2 2 (3x) (4y) 2 (4y) (5z) 2 (5z) (3x) = 9x2 16y2 25z2 – 24xy – 40yz 30zx (ii) (2a – 5b – 4c)2 = (2a)2 (5b)2 (4c)2 2 (2a) (5b) 2 (5b) (4c) 2Substitute x = u a to obtain f(u a) = a0 a1(u a) a2(u a)2 ⋯ Do some boring calculations, clear out things and express it as a power series in powers of u f(u a) = b0 b1u b2u2 ⋯ And now substitute back to x again f(x) = b0 b1(x − a) b2(x − a)2 ⋯Expand Using the Binomial Theorem (XY)^4 Use the binomial expansion theorem to find each term The binomial theorem states Expand the summation Simplify the exponents for each term of the expansion Simplify each term Tap for more steps Multiply by Anything raised to is



Ex 2 5 4 Expand Each Of The Following Using Suitable



If Number Of Terms In The Expansion Of X 2y 3z N Are 45 Th
Insert x n1 y next to the second number of Pascal's Triangle and add a "" sign 1x 5 5 x 4 y 10 10 5 1 ; · The first answer is WRONG It is a very common mistake to think that (xy)^2 equals x^2y^2, which it does not The distributive property only works for multiplication The correct way to expand (xy)^2 is to multiply (xy) (xy) You multiply each term in the first set of parentheses by each term in the second parenthesesExpand using identities (x2y4z)^2 Ask questions, doubts, problems and we will help you menu myCBSEguide Courses CBSE Entrance Exam Competitive Exams ICSE z = x 2 4y 2 4xyz 2 (2x4y)z = x 2 4y 2 z 2 4xy2xz4yz 1 Thank You ANSWER Related Questions Give figure twins of X is 30 degree less than y then the value of x and y are



A Maths I Rev 11 May



Be Able To Expand Brackets And Simplify The Expressions That Arise Ppt Download
X^2y^2z^2=4y2z(1) Now rearranging the terms we get x^2y^24yz^22z=0 Now we have to make them perfect square x^2y^24y44z^22z11=0 Then We get x^2(y2)^2(z1)^25=0 Or x^2(y2)^2(z1)^2=5 Therefore it is a sphere having centre (0,2,1) and radius 5^05 · this can then be written as (x^2 2xy y^2) (x^2 2xy y^2) and expand that out now to be 1x^4 (2xy) (x^2) x^2y^2 (2xy) (x^2) 4x^2y^2 (2xy) (y^2) (y^2) (x^2) (y^2) (2xy) 1y^4 now you just combine like terms and you get your answer 1x^4 (4x^3)y 6x^2y^2 4xy^3 1y^4Checklist Ready to be marked ?



Expand 4a 2b 3c 2



Square Algebra Wikipedia
Which means we are first going to multiply the x in the left hand bracket with everything in the right hand bracket, then we will multiply the 4 with everything in the right hand bracket So we will do x multiply x, x multiply 2, 4 multiply x, 4 multiply 2 Which comes out to be, x^2 2x 4x 8, FInally, simplying all the terms, the equationThanks Solve for x and y and z for the following x plus y plus z equals 9 xSubstitute x for a, 2y for b and z for c (x 2y z) 2 = x 2 (2y) 2 z 2 2(x)(2y) 2(2y)(z) 2(x)(z) (x 2y z) 2 = x 2 4y 2 z 2 4xy 4yz 2xz So, the expansion of (x 2y z) 2 is x 2 4y 2 z 2 4xy 4yz 2xz a minus b plus c Whole Square Formula To get formula / expansion for (a b c) 2, let us consider the



Solve D 2 3d 2 Y E E X Using Method Of Variation Of Parameters Mathematics Stack Exchange



Expand X 2 Y 1 4 2 Brainly In
Download free PDF of best NCERT Solutions , Class 8, Math, CBSE Algebraic Expressions and Identities All NCERT textbook questions have been solved by our expert teachers You can also get free sample papers, Notes, Important QuestionsExpand equations stepbystep full pad » x^2 x^ {\msquare} \log_ {\msquare} \sqrt {\square} \nthroot \msquare {\square} \le \geAtoZmathcom Homework help (with all solution steps), Online math problem solver, stepbystep



Expand 2x 3y Square Brainly In



4 Partial Derivatives And Their Applications Derivative Variable Mathematics
Expand polynomial (x3)(x^35x2) GCD of x^42x^39x^246x16 with x^48x^325x^246x16;If th equestion meant (xyz)^2The expansion is(xyz)^2= x^22xyy^22yzz^22zx Can you help with this equation?آلة حاسبة للتوسيع والتبسيط وسّع وبسّط التعابير الجبريّة خطوة بخطوة



X Square Minus Y Square Whole Cube Y Square Minus Z Square Whole Cube Z Square Minus Y Square Brainly In



Complex Number Wikipedia
Simplify (3x 4 y 7 z 12) 5 (–5x 9 y 3 z 4) 2 0 Who cares about that stuff inside the square brackets?Y ℓ m ( r ) {\displaystyle Y_ {\ell }^ {m} ( {\mathbf {r} })} , are known as Laplace's spherical harmonics, as they were first introduced by Pierre Simon de Laplace in 17 These functions form an orthogonal system, and are thus basic to the expansion of a general function on the sphere as alluded to aboveQuotient of x^38x^217x6 with x3;



Ex 2 5 4 Expand Each Of The Following Using Suitable



Ex 2 5 4 Expand Each Of The Following Using Suitable
מחשבון הרחבה ופישוט הרחב ופשט ביטויים אלגברים צעד אחר צעדWhen we have a sum (difference) of two or three numbers to power of 2 or 3 and we need to remove the brackets we use polynomial identities (short multiplication formulas) (x y) 2 = x 2 2xy y 2 (x y) 2 = x 2 2xy y 2 Example 1 If x = 10, y = 5a (10 5a) 2 = 10 2 2·10·5a (5a) 2 = 100 100a 25a 2(xy)^4 = x^4y^4z^44x^3y4xy^34y^3z4yz^34z^3x4zx^36x^2y^26y^2z^26z^2x^212x^2yz12xy^2z12xyz^2 Note that (ab)^4 = a^44a^3b6a^2b^24ab^3b^4 So we can find the terms of (xyz)^4 that only involve 2 of x, y, z by combining the expansions of binomial powers, One way to see that is to think about setting each of x, y, z



Simplifying Square Root Expressions Video Khan Academy



Example 7 Find Coefficient Of X6y3 In Expansion X 2y 9
Two examples involving binomial expansion Includes Pascal's triangle, combinations and moreSqrt z ^2 is not automatically converted to z Sqrt a b is not automatically converted to Sqrt a Sqrt b These conversions can be done using PowerExpand , but will typically be correct only for positive real argumentsIf None, an ordinary eigenvalue problem is solved (currently supported only if the base ring of self is RDF or CDF) OUTPUT For each distinct eigenvalue, returns a list of the form (e,V,n) where e is the



Systems Of Equations With Elimination X 2y 6 4x 2y 14 Video Khan Academy



2x 3 Y 3z 5 X 2y Z 4 3x Y 2z 3 Youtube
In elementary algebra, the binomial theorem (or binomial expansion) describes the algebraic expansion of powers of a binomialAccording to the theorem, it is possible to expand the polynomial (x y) n into a sum involving terms of the form ax b y c, where the exponents b and c are nonnegative integers with b c = n, and the coefficient a of each term is a specific positiveRemainder of x^32x^25x7 divided by x3; · Ok well first you must expand the whole problem so you multiply 2(x1)(x4) to expand and (x2)(x2) to expand the square you then combine like terms and then factor it you are going to get both and make them =0 so after you get your answer



Mathematics Notes



Solve 2x 3y 11 And 2x 4y 24 And Hence Find The Value Of M For Which Y Mx 3
Circle on a Graph Let us put a circle of radius 5 on a graph Now let's work out exactly where all the points are We make a rightangled triangle And then use Pythagoras x 2 y 2 = 5 2 There are an infinite number of those points, here are some examplesAlgebra Expand (xyz)^2 (x − y z)2 ( x y z) 2 Rewrite (x−y z)2 ( x y z) 2 as (x−yz)(x−yz) ( x y z) ( x y z) (x−y z)(x−yz) ( x y z) ( x y z) Expand (x−yz)(x−yz) ( x y z) ( x y z) by multiplying each term in the first expression by each term in the second expressionUsing algebra to solve 4(x 3) 3(x 2)Firstly multiply through to get rid of brackets and add up the terms



Pdf Extended Newton Raphson Method
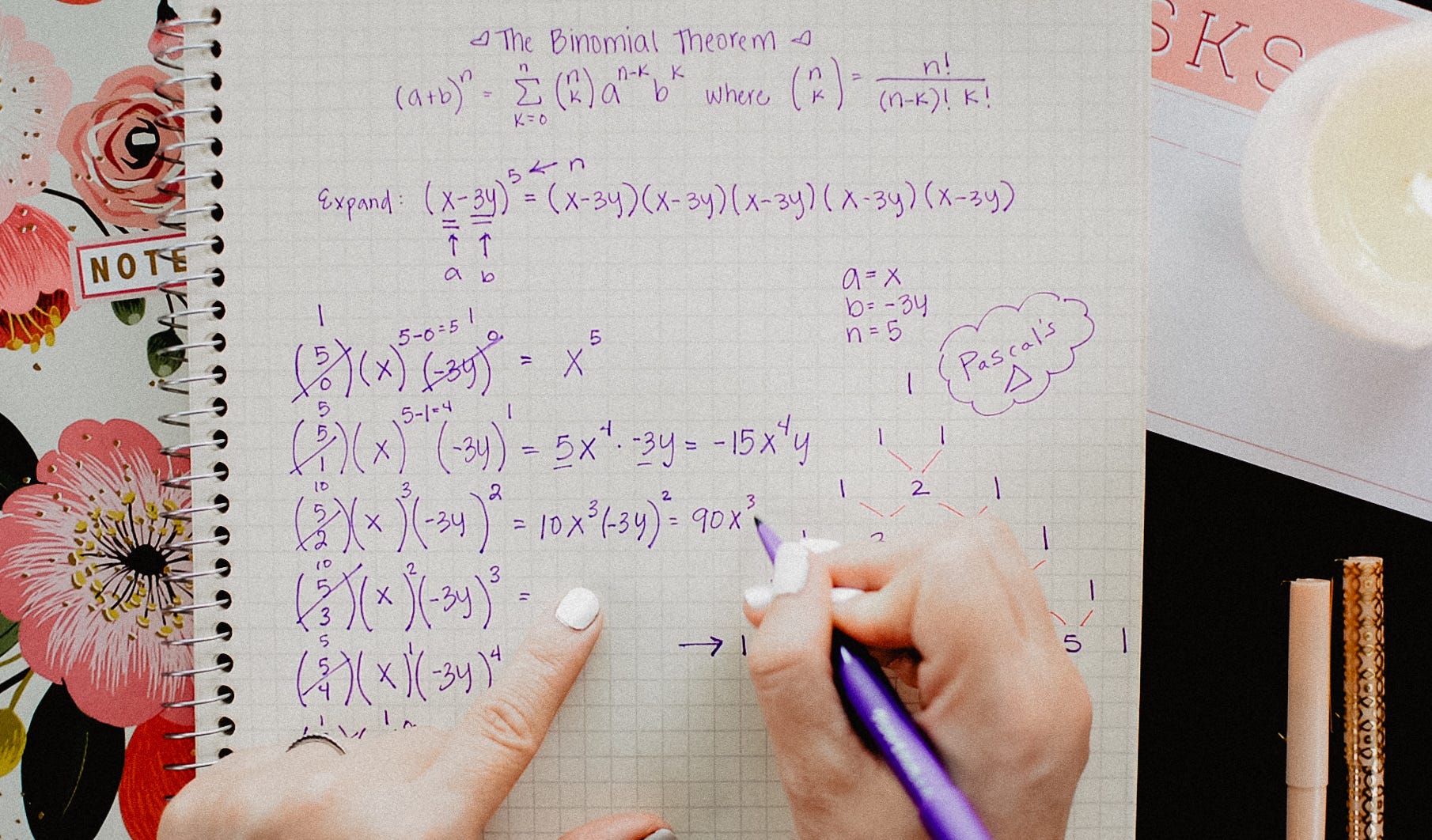


The Binomial Theorem Explained With A Special Splash Of Pascal S By Brett Berry Math Hacks Medium
4 Binomial Expansions 41 Pascal's riTangle The expansion of (ax)2 is (ax)2 = a2 2axx2 Hence, (ax)3 = (ax)(ax)2 = (ax)(a2 2axx2) = a3 (12)a 2x(21)ax x 3= a3 3a2x3ax2 x urther,F (ax)4 = (ax)(ax)4 = (ax)(a3 3a2x3ax2 x3) = a4 (13)a3x(33)a2x2 (31)ax3 x4 = a4 4a3x6a2x2 4ax3 x4 In general we see that the coe cients of (a x)nView more examples » Access instant learning tools Get immediate feedback and guidance with stepbystep solutions and WolframX 2 y 2 = z 2 Subtract y^ {2} from both sides Subtract y 2 from both sides x^ {2}=z^ {2}y^ {2} x 2 = z 2 − y 2 Take the square root of both sides of the equation Take the square root of both sides of the equation x=\sqrt {\left (zy\right)\left (yz\right)} x=\sqrt {\left (zy\right)\left (yz



Pdf Electric Potential Of A Uniformly Charged Square On Its Plane
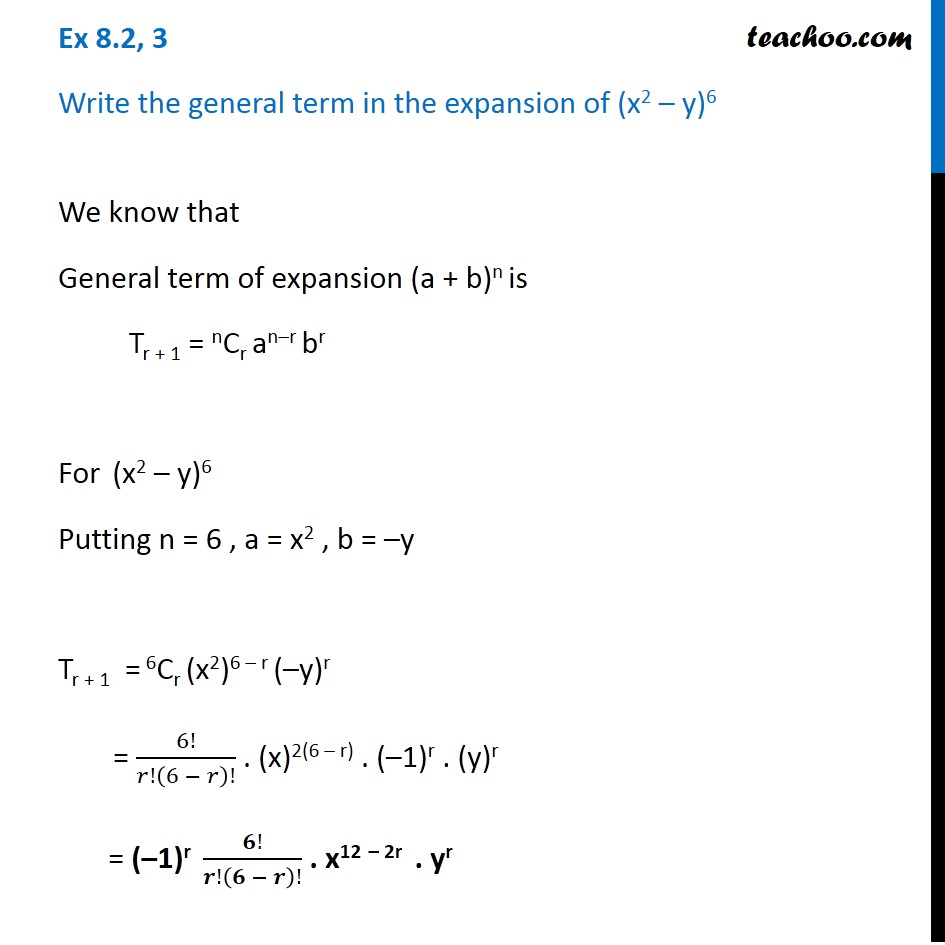


Ex 8 2 3 Write General Term In X2 Y 6 Chapter 8 Ex 8 2
Continue this process decrementing the power of x and incrementing the power of y until you place the term y n next to the final number 1x 5 5 x 4 y 10x 3 y 2 10x 2 y 3 5xy 4 1y 5 Exercises Expand (2x y) 4 (x y) 6Expand(S,Name,Value) uses additional options specified by one or more namevalue pair argumentsFor example, specifying 'IgnoreAnalyticConstraints' as true uses convenient identities to simplify the inputEigenvectors_left (other = None) ¶ Compute the left eigenvectors of a matrix INPUT other – a square matrix \(B\) (default None) in a generalized eigenvalue problem;



Rules Of Exponents



Graph X 2 Y 2 4 Youtube
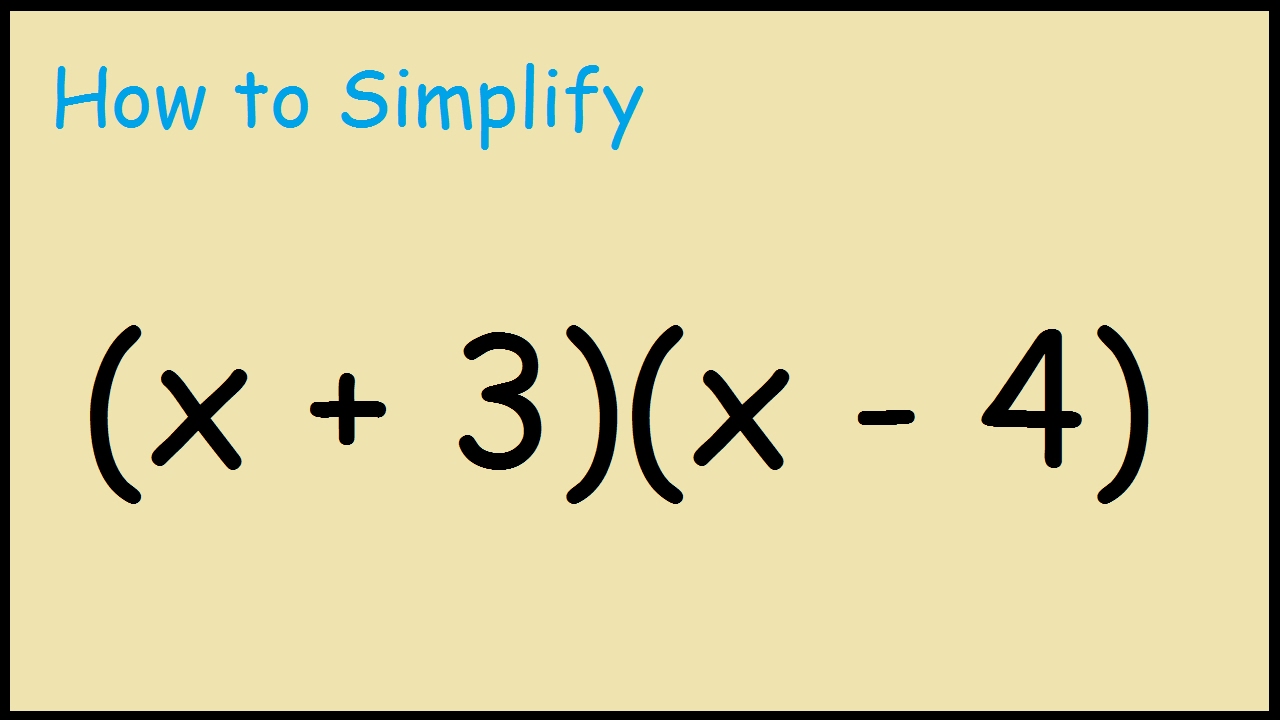


How To Expand X 3 X 4 Binomials Youtube



Expand 2x 3y 4z 2 Brainly In



Zaryal Teaching Academy Kpk Facebook



Expand Using Identity 2x 3y 3
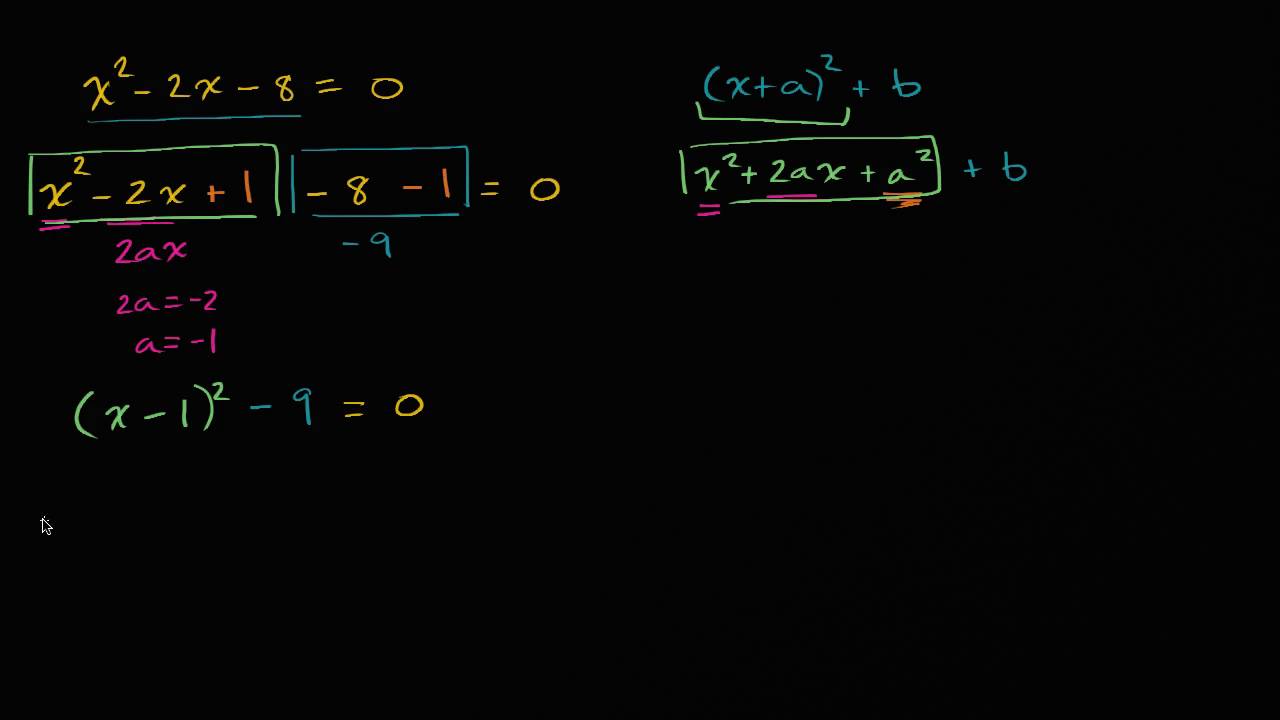


Worked Example Rewriting Solving Equations By Completing The Square Video Khan Academy
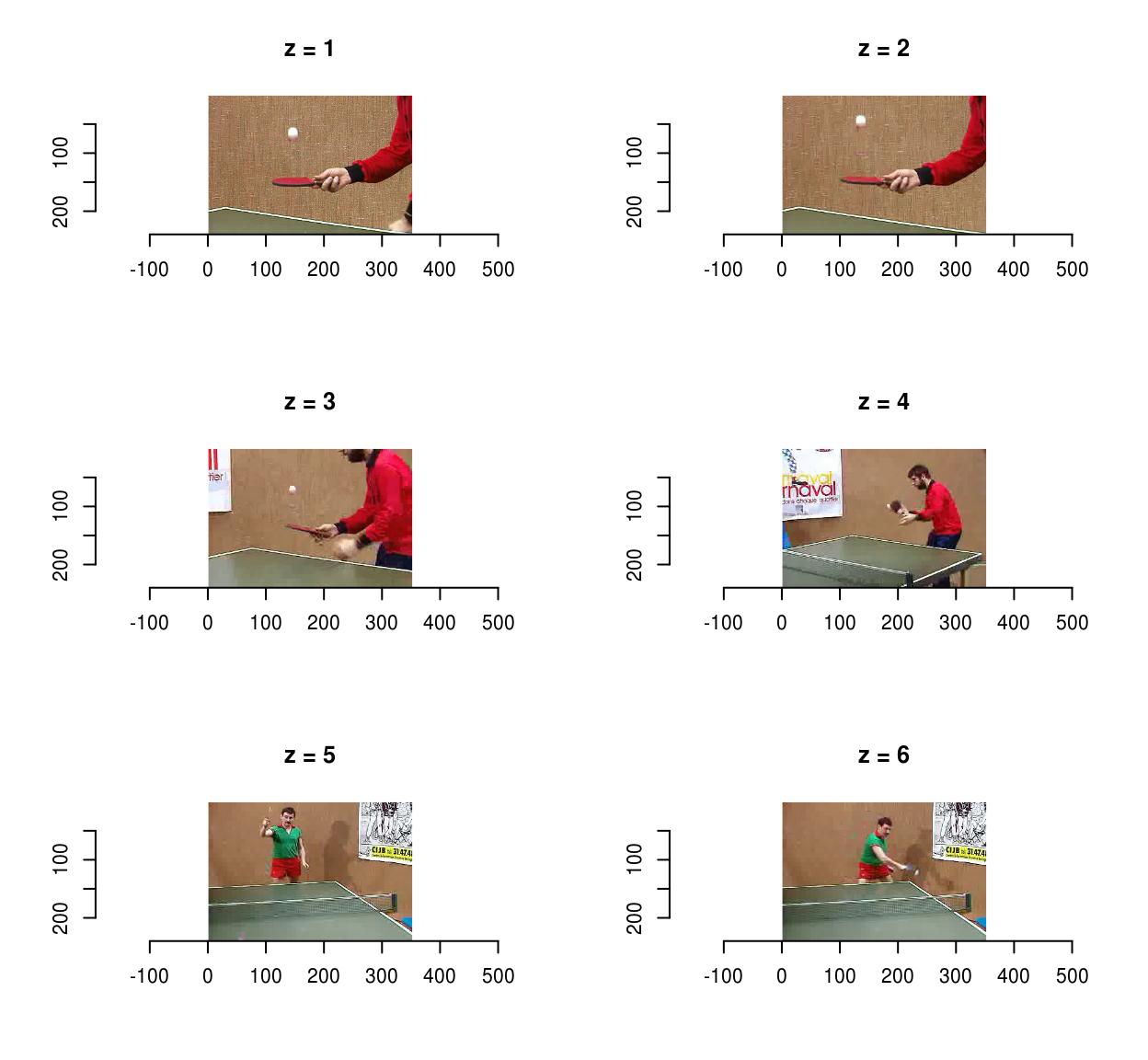


Imager An R Package For Image Processing



Example 7 Find Coefficient Of X6y3 In Expansion X 2y 9



Expand Using The Identity A 2x 3y Z 2 Brainly In



The Term Independent Of X In Expansion Of X 1 X 2 3 X 1



The Factors Of X 2 4y 2 4y 4x Y 2x 8 Are A X 2y 4 X 2y 2 B X Y 2 X 4y 4 C Youtube



Factorise 2x 2 Y 2 8z 2 2 2xy 4 2yz 8xz Brainly In



Factorise 25 X Square Y Square 9 Z Square 10 X Y 6 Y Z 30 X Z Brainly In
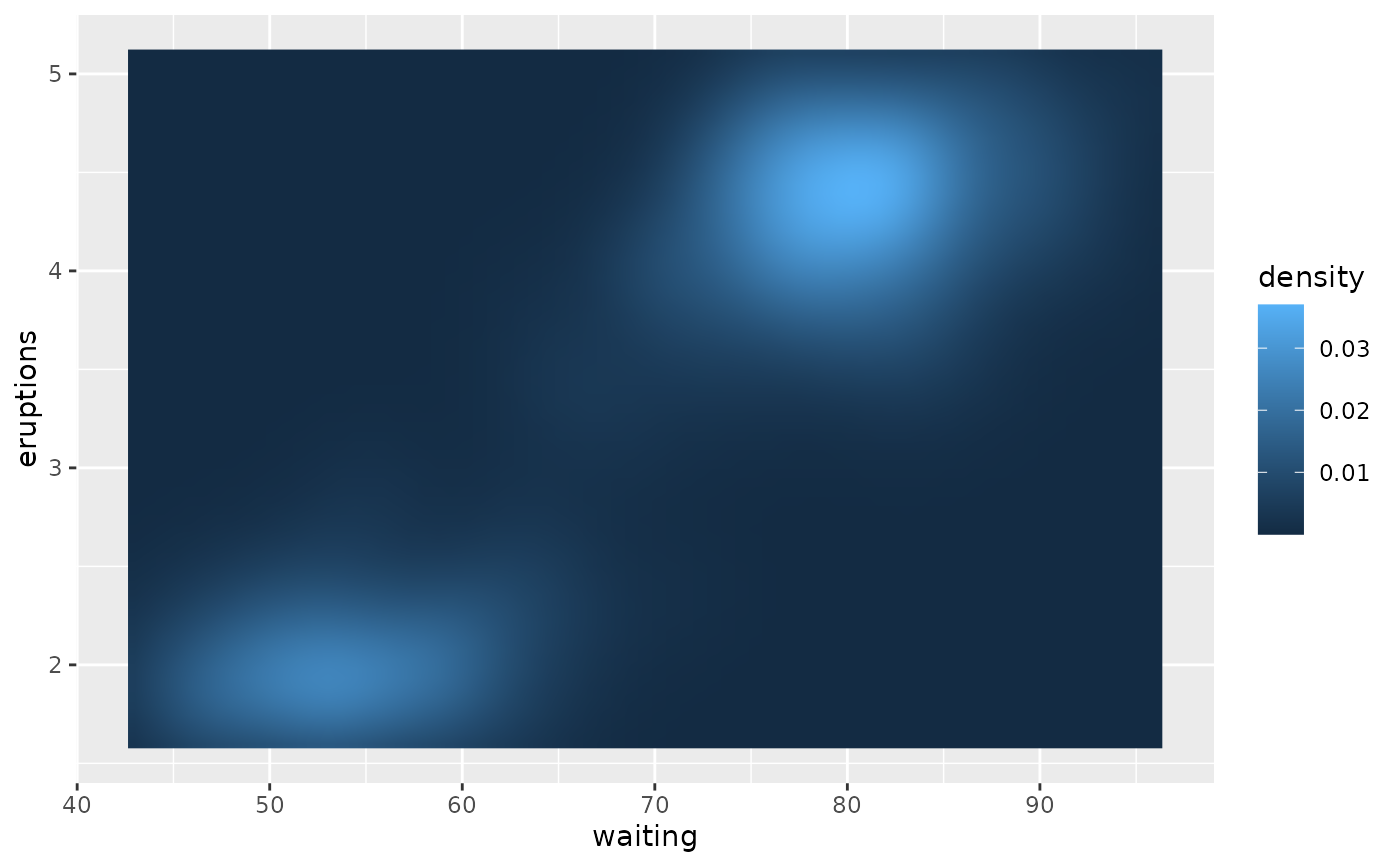


Rectangles Geom Raster Ggplot2



Partial Fraction Expansion Repeated Factors Video Khan Academy



Rd Sharma Class 9 Chapter 4 Algebraic Identities Free Pdf



Ex 2 5 4 Expand Each Of The Following Using Suitable



Example 19 Write 3a 4b 5c 2 In Expanded Form Examples
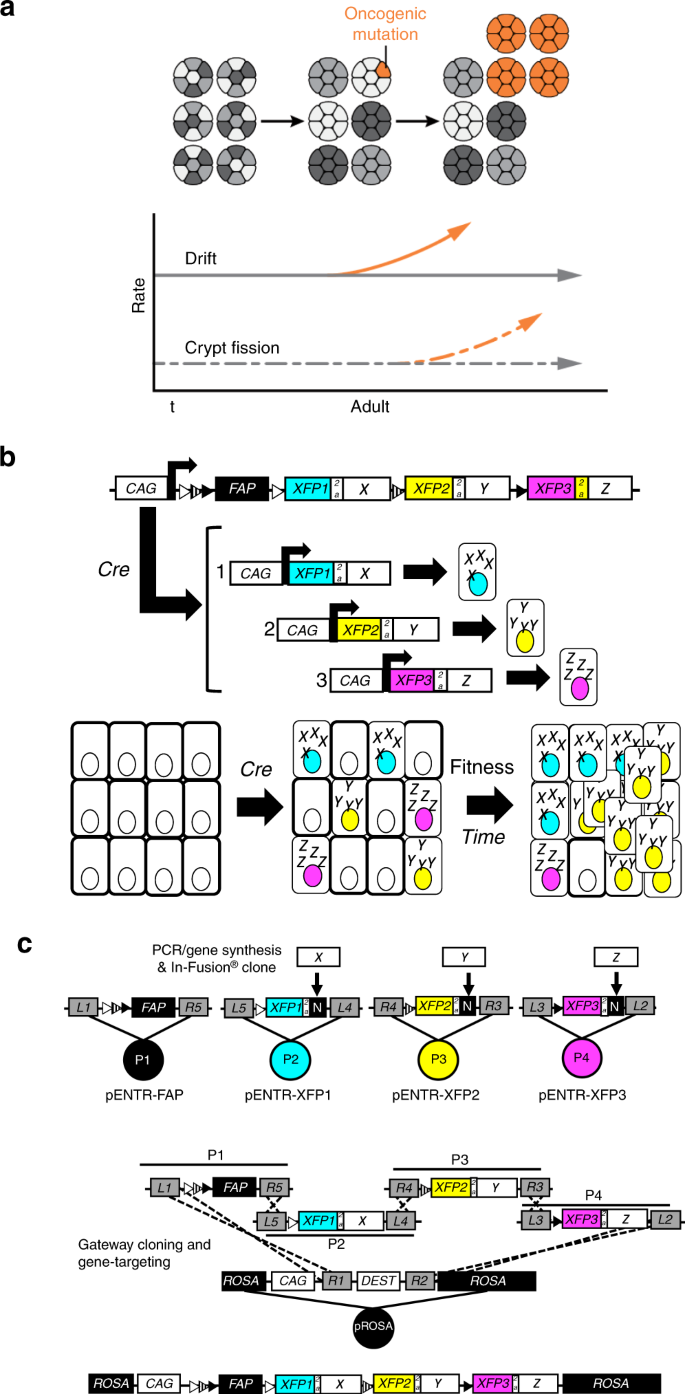


A Cancer Rainbow Mouse For Visualizing The Functional Genomics Of Oncogenic Clonal Expansion Nature Communications



Postmitotic Expansion Of Cell Nuclei Requires Nuclear Actin Filament Bundling By A Actinin 4 Embo Reports



Solved Question No 1 3 3 4 I Without Expansion Show T Chegg Com



Numbers In Python Real Python



Expand Each Of The Following Using Suitable Identities I X 2y 4z Square Brainly In



Example 21 Factorize 4x2 Y2 Z2 4xy 2yz 4xz Examples
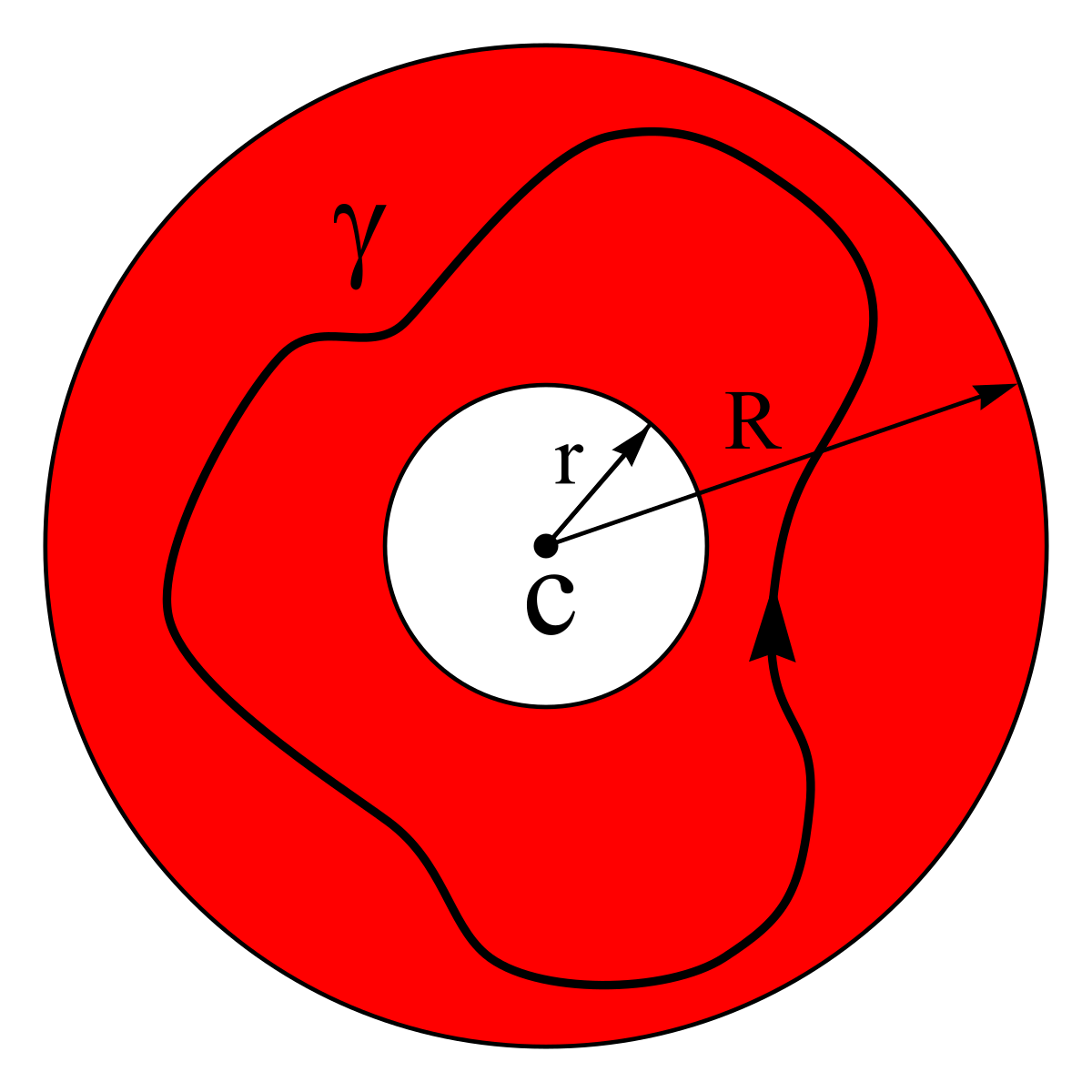


Laurent Series Wikipedia


If Z Is A Complex Number Then Is Z 2 Equal To Mod Z 2 Quora



2 X Square Y Square 8 Z Square 2 Root 2 X Y 4 Root 2 Y Z 8 X Z Brainly In



Test The Consistency Of The System X Y 2z 1 X Y Z 3 X 3y 3z 1



Mathematics Notes


Covid Economics Centre For Economic Policy Research
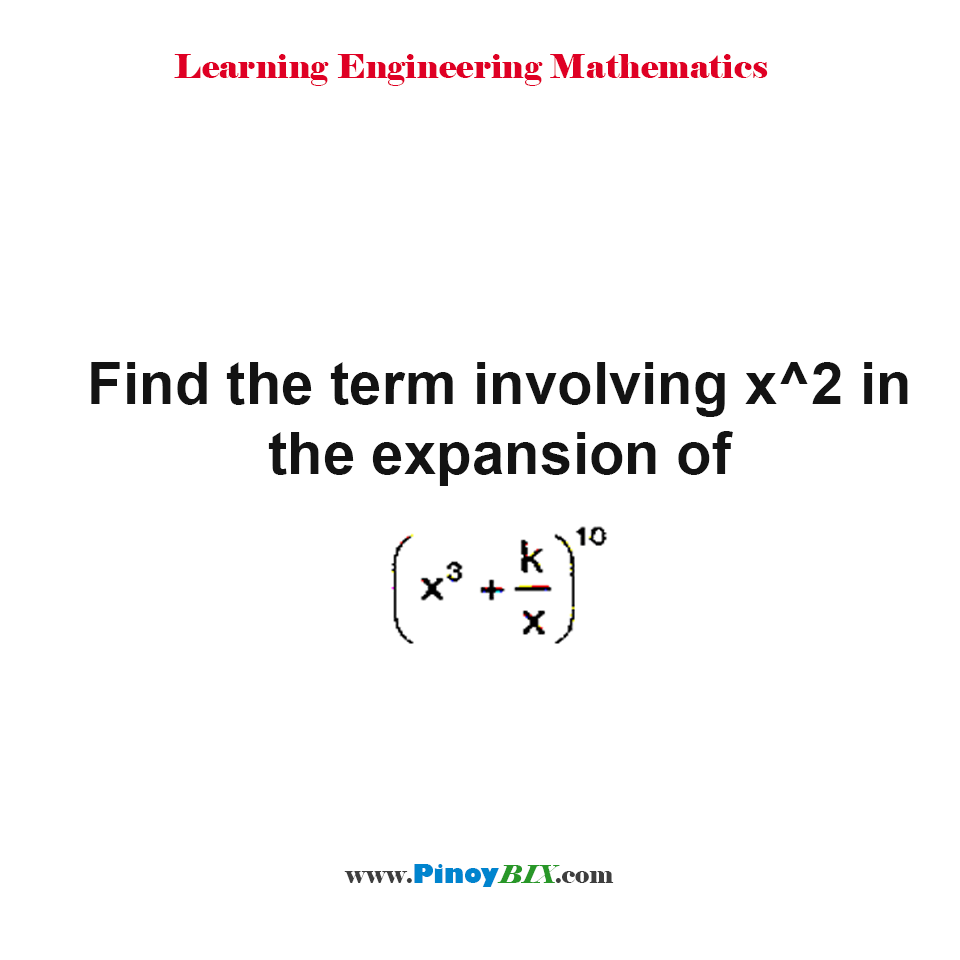


Solution Find The Term Involving X 2 In The Expansion Of X 3 K X 10
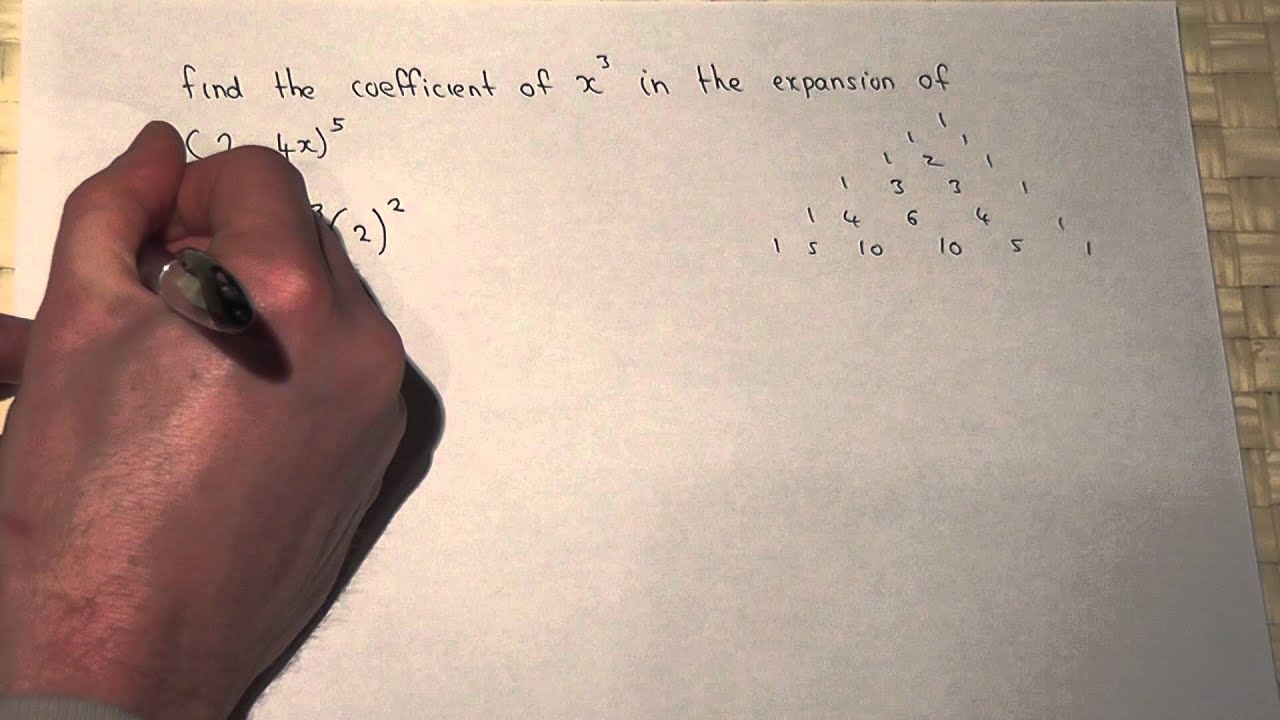


Binomial Theorem Finding The Coefficient Of X 3 In 2 4x 5 Youtube
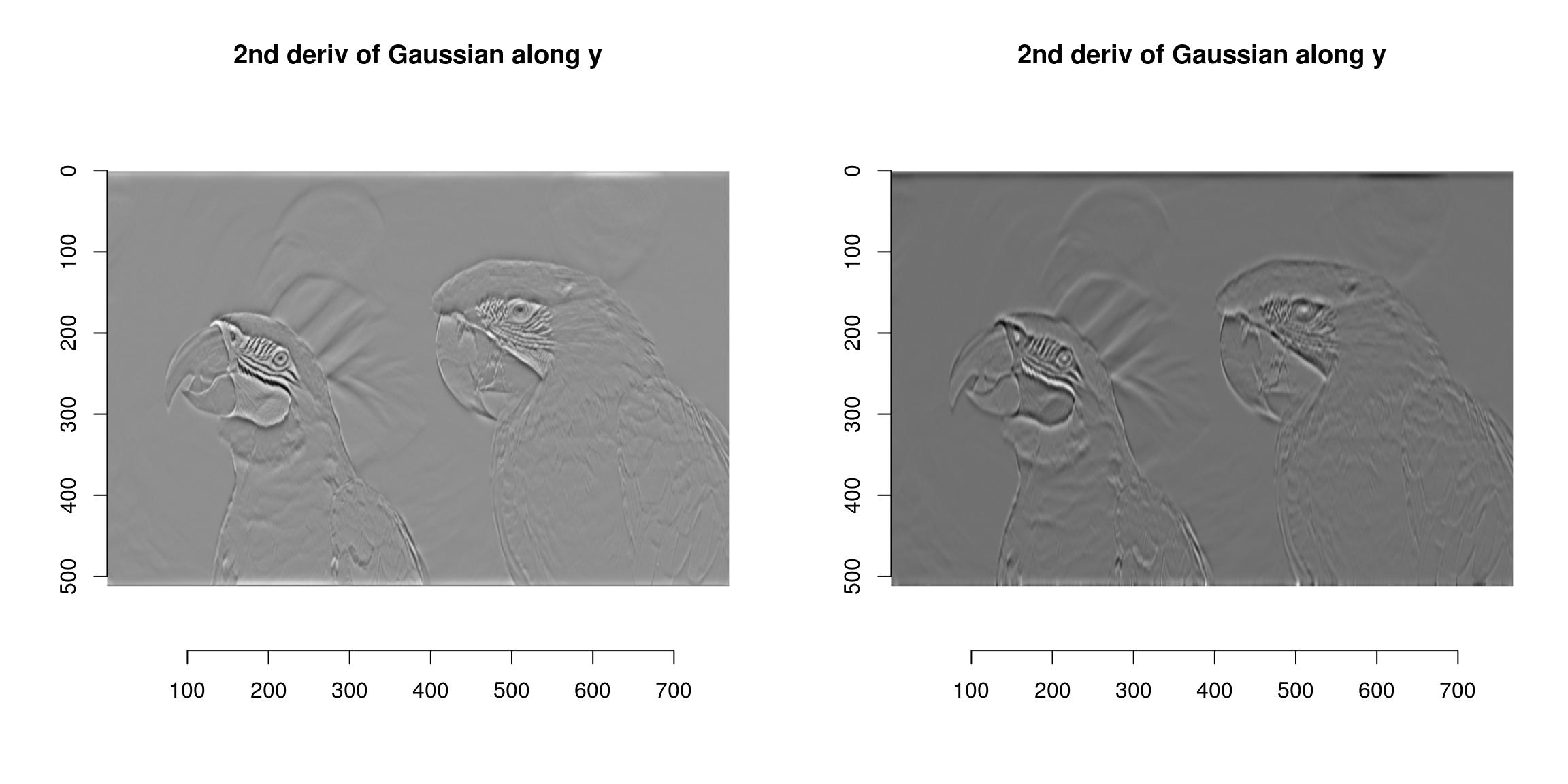


Imager An R Package For Image Processing



Find The Coefficient Of X 2 Y 3 Z 4 In The Expansion Of Ax By
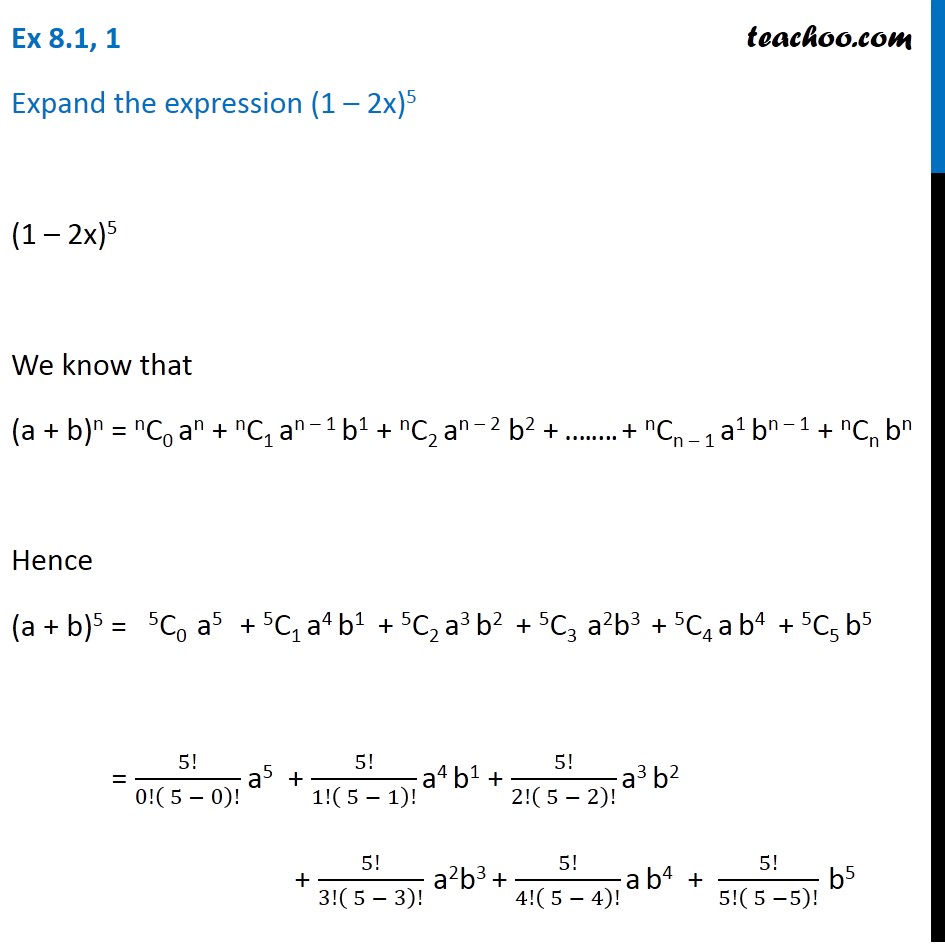


Ex 8 1 1 Expand 1 2x 5 Chapter 8 Class 11 Binomial



How To Expand Using The Identity A B C 2 B2 C2 2ab 2bc 2ca Youtube


Spherical Harmonics Wikipedia



Question Video Finding The Equation Of The Tangent Plane To A Surface At A Given Point Nagwa



Algebra Using Brackets Ppt Download



Expand And Simplify Binomial Squares 2x 3y 2 Youtube



Selina Mathematics Class 9th Solutions Icse Chapter 4 Expansion
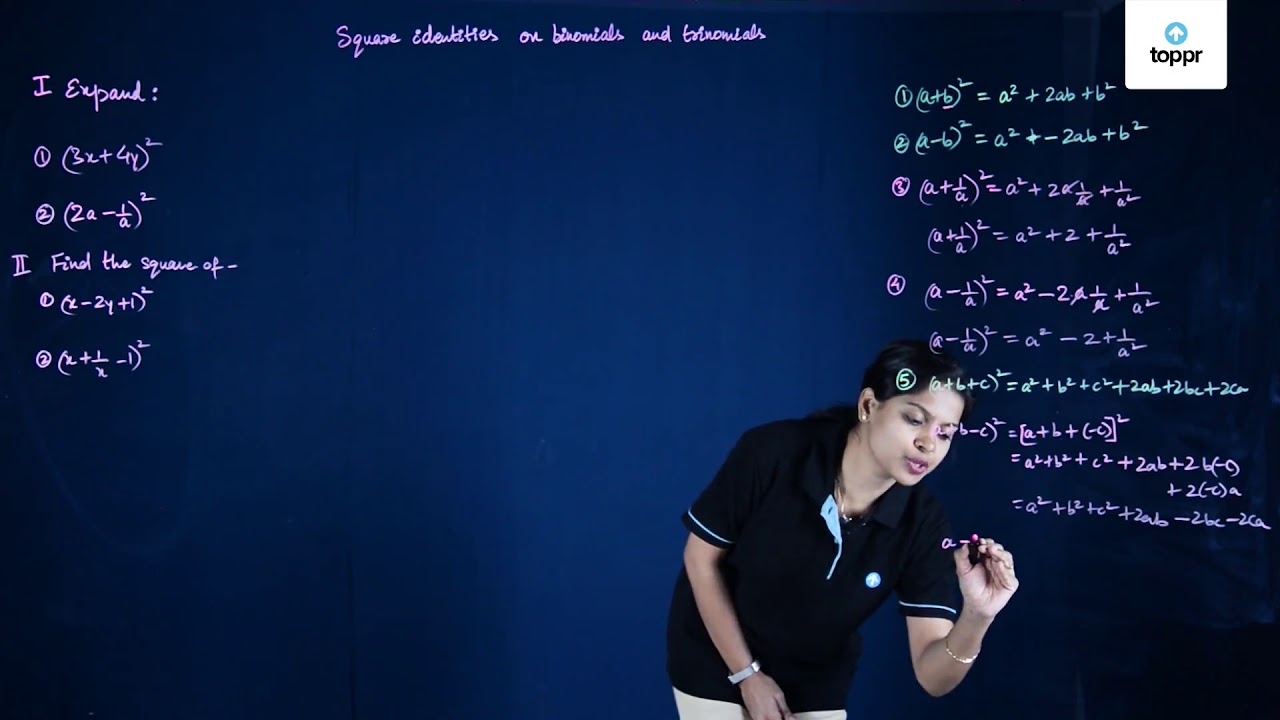


Standard Identities Of Binomials And Trinomials Equations Examples



0 件のコメント:
コメントを投稿